Jordi Vila received a Bachelor’s double degree in Mathematics and Aerospace Engineering from Centre de Formació Interdisciplinària Superior (CFIS, UPC), and a Master in Advanced Mathematics and Mathematical Engineering from Facultat de Matemàtiques i Estadística (UPC) in 2016 and 2017, respectively. From October 2017, he is a PhD candidate in Prof. Huerta’s research group, where he focuses on high and low order methods for compressible fluid flows.

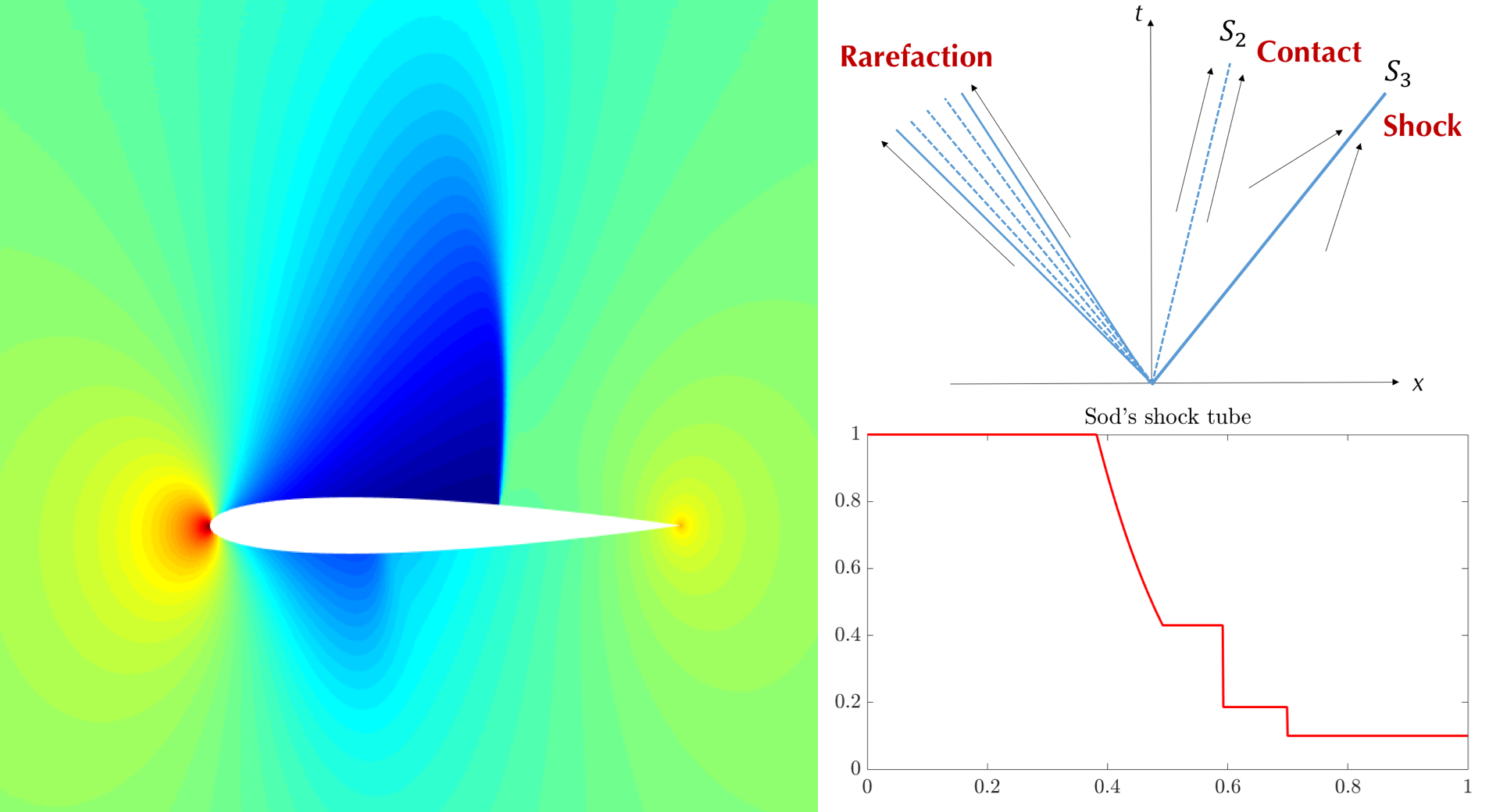
The equations modelling compressible fluid flows, when the effects of viscosity and heat transfer are neglected, reduce to the Euler equations. This hyperbolic system of PDEs admits solutions describing a complex phenomenona, such as rarefactions, shocks and contact waves.

Riemann solvers are a family of numerical methods widely used to describe, either exactly or by means of an accurate approximation, the wave structure of these types of solutions. In this talk, some of the most common approximate Riemann solvers for the Euler equations will be reviewed in the context of the finite volume method. Numerical experiments will be presented to compare the discussed strategies, focusing on their ability to accurately describe each kind of wave.