Jordi Vila received a Bachelor’s double degree in Mathematics and Aerospace Engineering from Centrede Formació Interdisciplinària Superior (CFIS, UPC) and a Master in Advanced Mathematics andMathematical Engineering from Facultat de Matemàtiques i Estadística (UPC) in 2016 and 2017, respectively. From October 2017, he is a PhD candidate in Prof. Huerta’s research group where he focuses on high and low order methods compressible fluid flows.

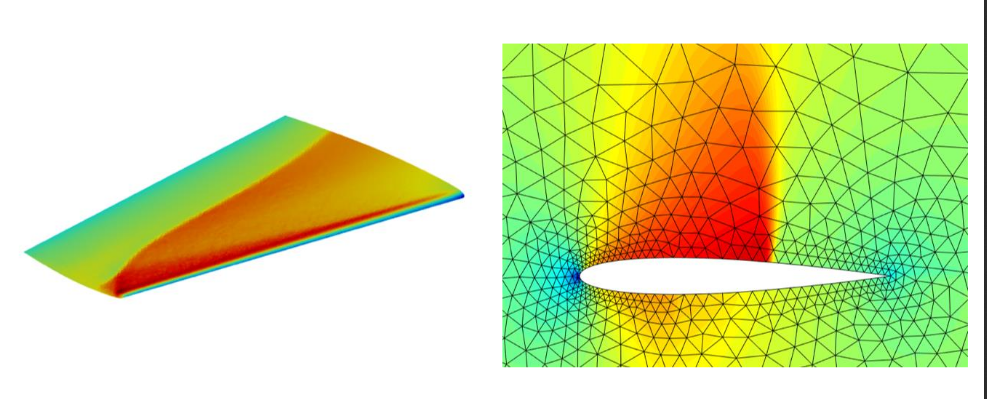
The numerical simulation of compressible flows has become a tool of major relevance for many applications in the aerospace industry, where the arising flow phenomena are subject to significant changes in fluid density due to high operating velocities. Because of the spatial discretisation of these conservation laws –such as the compressible Euler equations–, a set of Riemann problems emerge in the entire computational domain. The numerical solution of these equations requires the introduction of Riemann solvers, which are highly responsible of the accuracy of the method. The talk will address the use of Riemann solvers in the context of hybridised discontinuous Galerkin methods; namely, their implicit implementation within the numerical fluxes. Different types of Riemann solvers (Lax-Friedrichs, Roe and Harten-Lax-van Leer-Einfeldt) will be introduced. In order to show the potential of Riemann solvers to capture complex flow phenomena such as expansions or shock waves, different examples, including both academic and large-scale simulations of industrial problems, will be presented.